I don’t know why—if I thought as I did that the Beirut blast on 4 August 2020 was nuclear—that I absolutely had not thought about whether that blast had left a crater in the first few days after the blast.

Back in December 2018 I learned about the work of Dimitri Khalezov and Joe Vialls who argued that truck bombs, blamed for decades for massive explosions that had killed thousands and wreaked tremendous damage—simply did NOT blast large craters, I then started looking for evidence of craters following “aboveground explosions” like “truck bombs” as evidence that these aboveground explosions might be nuclear events.

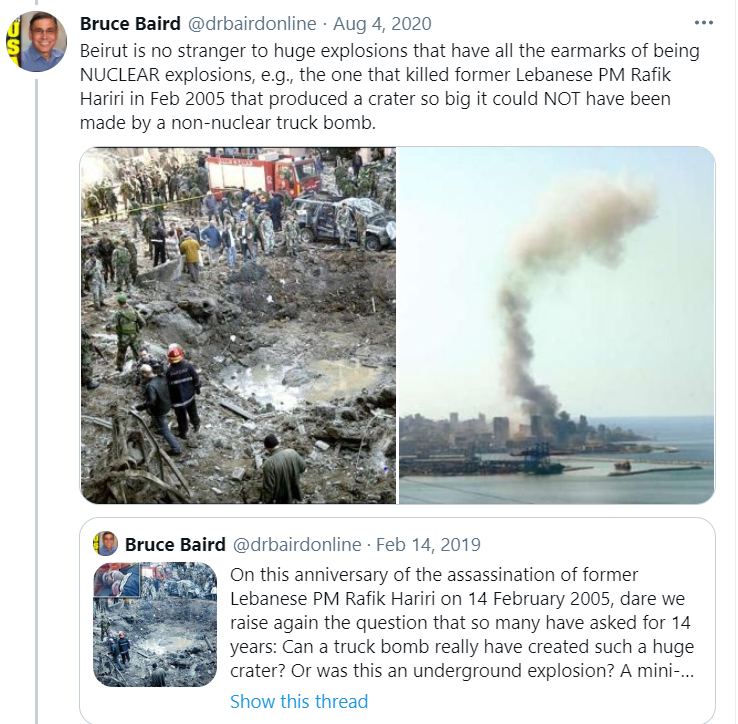
Indeed on August 4th, the day of the 2020 Beirut explosion, I had tweeted that the crater 8.3-11.4 meters in diameter with height 1.9 meters left after February 2005 assassination of former Prime Minister Rafik Hariri suggested that this was a nuclear explosion.

 

But maybe because there was no mention of any truck bomb and I didn’t see (or at least I don’t remember seeing) any photos of a crater in the first few days after the 2020 explosion, I just didn’t think about craters.

But, even if I didn’t see them, there were, in fact, reports about a massive crater—much, much bigger than the 2005 Hariri crater—on August 4th, the day of the explosion. Indeed, Bellingcat—whose tweet “We can, however, state with confidence that this was not a nuclear blast” I already noted in Chapter 1—on August 4 posted a detailed article on their website, an article that I actually tweeted on August 8 but apparently did not bother to read!

When are all those brave souls who rushed to challenge the "official" chemical weapons story in Syria going to get up & challenge the "official" ammonium nitrate warehouse story in Beirut? Right now they're all on the side of Bellingcat!https://t.co/K0oVeHgBzZ

— Bruce Baird (@drbairdonline) August 8, 2020

In the August 4th Bellingcat article titled “What Just Blew Up In Beirut?”, Nick Waters reported that “Images from the next day show that the port has been utterly devastated. The location of the warehouse is now simply a vast crater, approximately 150 meters wide, filled with water.” Wow! If I had seen that the Beirut explosion had blasted a 150-meter wide crater, I would have known for sure, based on what I had learned over the past year about cratering, that this explosion could only have been nuclear. ¹

On August 5th, CNN reported based on their measurement of the satellite imagery of the explosion site using geospatial software, they calculated a slightly smaller crater, roughly 124 meters (or about 405 feet) in diameter. They claimed the assessment was accurate within 10 meters ² But again, if I saw the article, it did not really register with me.

In the days after the explosion—based on my experience as a chemical engineer and a cursory reading of the literature—I did challenge in a few replies the idea that sacks of ammonium nitrate stacked on the floor of a warehouse could have possibly caused such an explosion or blasted a large crater but I didn’t launch an entire new thread on the subject.

All the standard reference books say "Only under extreme conditions of heat and pressure in a confined space will ammonium nitrate explode." That's what happened at Texas City in 1947. But I doubt seriously that's what happened at Beirut two days ago.https://t.co/SkzvItIdHg pic.twitter.com/QhsvvrVOpF

— Bruce Baird (@drbairdonline) August 6, 2020

 

Even if the warehouse was on landfill & crater only 120m, the (highly unlikely) explosion of 2750 tonnes of ammonium nitrate piled on a warehouse floor could NOT have blasted such a crater! I think I need to update this "truck bomb" thread for warehouses!https://t.co/pxDUcuZsQo

— Bruce Baird (@drbairdonline) August 8, 2020

 

I need to update this "truck bomb" thread to cover warehouses b/c despite all the so-called "science" you can read online, it is PHYSICALLY IMPOSSIBLE for 2750 tonnes of bags of NH4NO3 sitting on the floor of this warehouse to blast that Beirut crater!https://t.co/G9KHzBccmV

— Bruce Baird (@drbairdonline) August 8, 2020

There are so many things wrong with believing an arrangement of bags of NH4NO3 on the floor of a warehouse could blast a large crater that it really needs an entire thread. In SURFACE blast (HOB=0), the device is half buried. HOB is from surface to center of blast. pic.twitter.com/OcOGpemGRS

— Bruce Baird (@drbairdonline) August 8, 2020

What finally motivated me to launch a full thread on the impossibility of the “official” story was the widespread reporting across many different media outlets around the world on August 9th of an Agence France-Presse (AFP) report of a “Lebanese security official” citing assessments by “French experts on the scene” that the Beirut port blast crater is 43 meters deep! ³

 

On August 10, Wikipedia was quick to incorporate both the CNN crater diameter and AFP crater depth into their article on “2020 Beirut explosion.” ⁴

Within the port area, the explosion destroyed a section of shoreline and left a crater roughly 124 m (407 ft) in diameter and 43 m (141 ft) in depth.^{[114][115][116]}

 10:02, 10 August 2020‎ Dying talk contribs‎  101,551 bytes +343‎  →‎Damage: add details re crater size undo

 

Launching the Twitter Thread

With that report, taking all the knowledge I had gained in 2019 about nuclear explosions and cratering, I immediately launched what would be by far my biggest and most important twitter thread ever.

I decided to kick off the thread with a “teaser” in the hopes of attracting both people who question 9/11 as well as people curious about Beirut even though I had no intention of discussing 9/11 in the body of the thread. (Later I did have some contact with people interested in Beirut but turned off by my “conspiracy theory” of 9/11. But I’m sure such people would have thought that what I had to say about Beirut was “conspiracy theory” as well!) 

The kind of person who believes 2750 tonnes of ammonium nitrate stacked in bags on a warehouse floor blasted a 43-meter deep crater in Beirut is the kind of person who believes two jetliners caused the Twin Towers to "collapse" & destroyed the rest of WTC on 9/11!
Thread
1/ pic.twitter.com/nTr9EZc1gl

— Bruce Baird (@drbairdonline) August 9, 2020

Immediately I proceeded to draw upon my knowledge of nuclear tests and cratering to determine how big a crater one might expect from 2,750 tonnes of ammonium nitrate resting on the floor of a warehouse in the port of Beirut. Firstly, since all studies of cratering are based on the “equivalent explosive yield in tons of TNT,” we need to calculate the “equivalent explosive yield” of this amount of ammonium nitrate. I had already tweeted that I did not think ammonium nitrate on the floor of a warehouse would even explode, but for the sake of argument I chose to just go with the 1,155 “tons” of TNT reported by the New York Times.

NYTimes reports 2750 tons (tonnes?) of ammonium nitrate was "roughly equivalent to 1,155 tons of TNT" (assumes RE factor 0.42). Let's assume all that NH4NO3 could somehow be made to burst like a high explosive bomb. How deep a crater would you expect?
2/https://t.co/AtJs4aNMg5

— Bruce Baird (@drbairdonline) August 9, 2020

So how big a crater would 1,155 tons of TNT resting on the floor of a warehouse in the port of Beirut blast? Well, luckily we have a highly comparable precedent in a nuclear test (codename “Sugar”) performed at the Nevada Test Site (NTS) just north of Las Vegas in November 1951 as part of Operation Jangle sponsored by Los Alamos National Laboratory. For comparison purposes, here is a video of Jangle Sugar:

 

Sugar was mnemonic for “surface” because the fission Mk-6 bomb was detonated on the surface of the desert alluvia. Fortuitously, Sugar’s yield—1.2 kilotons of TNT equivalent—was very close to the 1.155 kilotons of TNT equivalent that the New York Times calculated for the 2020 Beirut “surface” explosion.

So how deep a crater did Jangle Sugar blast in desert alluvia? Would you believe only a depth of 17 feet (5.2 m) and diameter of 90 feet (27.4 m)—far, far less than the 141 feet (43 m) depth & 405 feet (124 m) diameter that MSM was reporting for Beirut for the same size “surface” explosion!

The details for Jangle Sugar and these other nuclear cratering tests come from Table 2 “Crater Parameters for Nuclear Cratering Events” compiled by Henry F. Cooper, Jr., Estimates of Crater Dimensions for Near-Surface Explosions of Nuclear and High-Explosive Sources published in September 1976 by Lawrence Livermore Laboratory. ⁵

Now at this point you may be wondering how much the difference in crater dimensions might have been due to the difference in media between the landfill at the Port of Beirut and the desert alluvium at NTS. Although the two media are certainly not identical, a study by the U. S. Army Engineer Waterways Experiment Station titled Cratering by Explosions: A Compendium and an Analysis published in January, 1974, has found comparable numbers for crater depth for all kinds of media from moist clay to hard rock, so one would expect, all other things being equal, no order-of-magnitude differences between the craters produced. ⁶

So if a surface 1.2-kt explosion can only blast a 5-meter deep crater, how could such an explosion possibly have blasted a 43-meter deep crater like Beirut?

Well in order to blast a larger crater you can generally do two things: 1) bury the explosive device deeper and/or 2) increase its explosive energy (TNT equivalence). For example, when Los Alamos increased the Depth of Burst (DOB) of the same 1.2-kt bomb to 17 feet below the ground (DOB = 17 feet) in the Jangle Uncle test (“Uncle” mnemonic code for “Underground”), the crater depth increased from 17 feet to 53 feet with the shape of the crater changing from a “Surface Burst” to “Shallow DOB” as shown in the figure below.

With Teapot Ess test in March 1955, Los Alamos buried the 1.2-kt device even deeper (DOB = 67 ft) and blasted a 90-ft deep crater as they moved closer to “Optimum DOB”, i.e., the biggest possible crater with that particular device.

Here’s a video of the Teapot Ess test:

 

To go deeper than the “Optimum DOB” ~ 67 ft, the 1.2-kt device starts to produce smaller and smaller craters until it can no longer blast a conventional crater.  At deeper levels, the result would look more like the “deeply buried crater” or “subsidence crater” pictured above. Thus there is NO WAY a 1.2-kt device could actually blast a 43-m (141-ft) deep crater, at least not in desert alluvium. The Beirut crater would have required a bigger explosion than 1.2 kilotons of TNT equivalent.

So how big an explosion would it have taken to blast a 43-m deep crater like that found in Beirut? Well that depends on how deep you want to bury the device. How about burying a 100-kt device 635 feet beneath the surface? That would produce WAAAY TOO BIG A CRATER! That is actually the result of Storax Sedan (July 1962) that, according to Wikipedia, produced “the largest human-made crater in the United States”  of 323 ft (98.4 m). ⁷

Here’s a video of Storax Sedan and a photo of what the crater looks like today from the observation deck:

 

Figure 4.3

Fortunately, for determining how big an explosion and/or how deep you would have to bury an explosive device to produce a certain size crater, the U. S. Army Engineer Waterways Experiment Station have made the job easy by compiling all depth results of cratering tests in the NTS desert alluvium & similar media in this declassified Figure 4.3 “Scaled crater depth versus HOB (DOB) for large explosions in desert alluvium and similar media” from a heavily redacted study Operation Sun Beam, Shots Little Feller I and II: Crater Size and Shape (March 1965) ⁸

 

Now, unless you were a math nerd in high school, most of you are probably going to roll your eyes looking at Figure 4.3. But with a little practice it is really not that hard to use. It is just an x-y coordinate graph—the same kind you worked with over and over again in high school algebra—where x = “Scaled Height (Depth) of Burst” and  y = “Scaled Crater Depth”.

Height of Burst (HOB) is used for aboveground explosions measured as the height of the center of the explosion (in feet) ABOVE the surface whereas Depth of Burst (DOB) is used for belowground explosions measured as the depth of the center of the explosion (in feet) BELOW the surface. HOB = 0 means the center of the explosion is exactly ON the surface which in general represents an explosive device half-buried in the earth. For an explosive device actually resting on the surface (e.g., Jangle Sugar), HOB is typically half of the height of the explosive device. Thus HOB for Jangle Sugar as shown in Table 2 (above) was actually +3.5 feet.

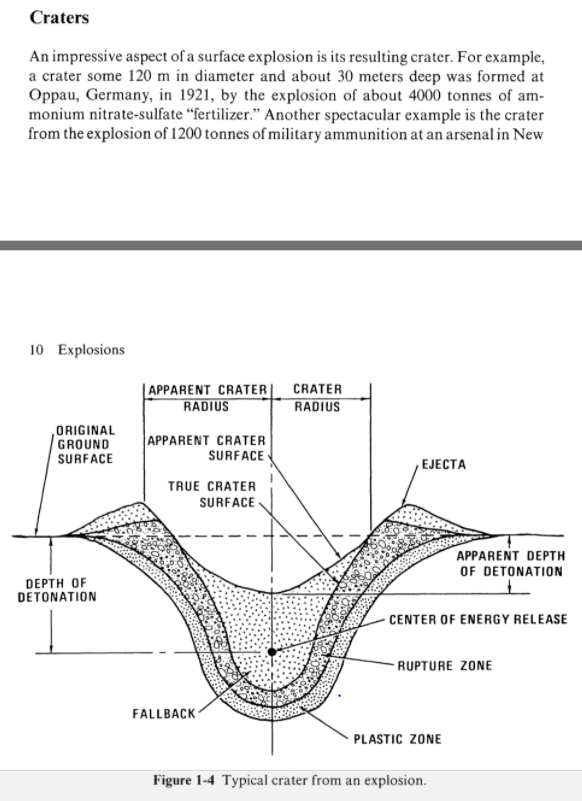
“Apparent crater depth” (as depicted in Figure 4.3) is shown in the diagram below as D_a (“apparent depth”)—the  distance between the original surface and the bottom of the “apparent crater boundary”, i.e., the bottom of crater after all the fallback has settled. Cratering studies almost always focus on “apparent crater depth” because the measurements are far easier to determine and correlations work just as fine (if not better) than “true crater depth.”

---

One rather strange aspect of using Figure 4.3 is the units used. Both x and y values are measured not in units of “feet” but  in units of “scaled feet” ft/kt^(1/3.4). That is, you have to “scale” the HOB/DOB and crater depth (measured in feet) by dividing by the actual explosive yield (measured in kilotons of TNT equivalent) raised to the 1/3.4 power. (To take the cube root of the explosive yield would be raising it to the 1/3 power while the fourth root would be to the 1/4 power, so 1/3.4 would lie in between the cube and fourth roots.)

Cratering studies have long shown that, if the HOB/DOB, crater depth, crater radius, and other aspects of cratering are properly scaled by dividing by the explosive yield raised to some fractional power, the results can then be plotted as smooth curves like Figure 4.3 that work for both relatively small and large explosions, conventional (i.e., non-nuclear) as well as nuclear explosions. Different studies have suggested different exponential values but they generally fall in the range between cube root (1/3) and fourth root (1/4). For the study on which Figure 4.3 is based, 1/3.4 was determined to produce the best fit.

Given two of the three variables (explosive yield, HOB/DOB, apparent crater depth), you can use Figure 4.3 to calculate the third variable. In the case of the 2020 Beirut blast, all we have is one variable (apparent crater depth = 43 meter), so we cannot find an exact solution but we can try various explosive yields and then determine how deeply the explosive device would have had to have been buried for each yield.

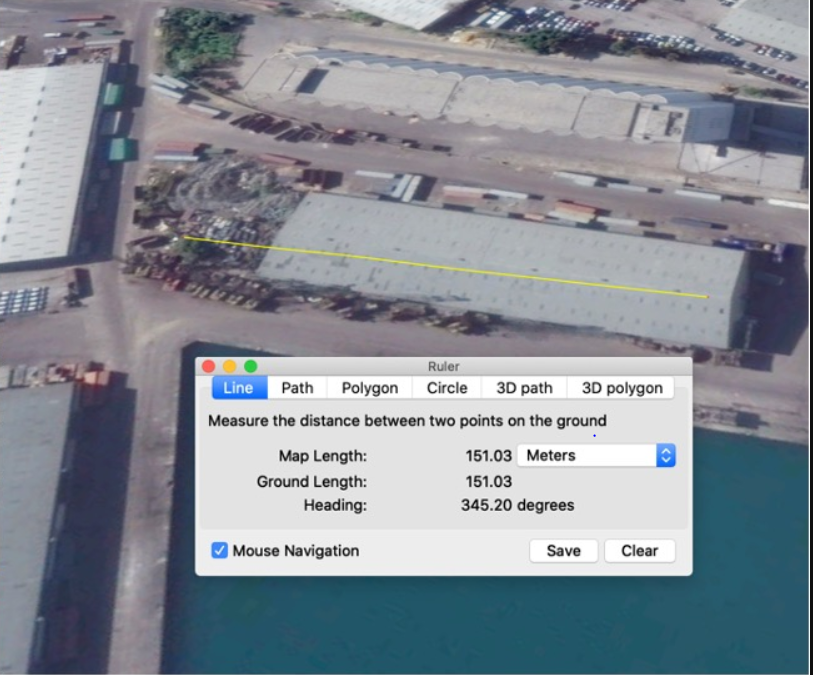
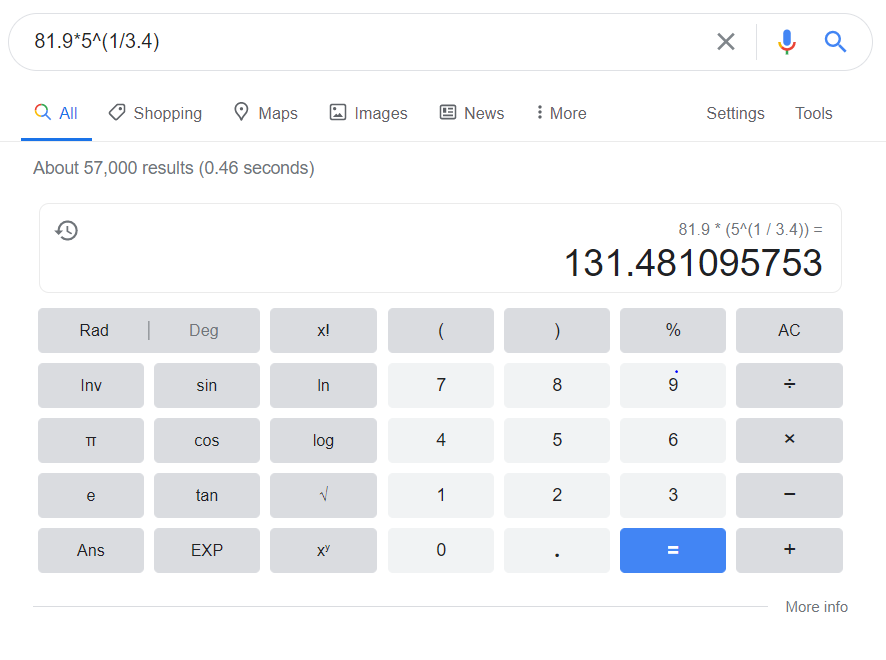
To demonstrate how Figure 4.3 can help us figure out what kind of explosion blasted the 2020 Beirut crater, we can start by calculating the smallest explosive yield that could have actually blasted a conventional crater with apparent crater depth of 43 meters (141 feet). By trial and error, I calculated this would have required an explosive yield of at least ~5 kilotons TNT equivalent. (The scaled crater depth for any smaller explosive yield falls above—and thus off—the curve of Figure 4.3 and when you calculate the crater depth for greater DOB the calculated depths fall short of 43 meters.) To calculate the DOB for 5 kt using Figure 4.3, you can actually just use your search engine to calculate the y-value (scaled crater depth) of 87.8:

I interpolate a scaled DOB (to the left of the peak point)

x = 81.9

Then plugging this value into the search bar, I convert scaled DOB into DOB and come up with a DOB of 131.5 feet (40.2 meters):

Using the same method, I came up with DOB for a 43m (141-ft) deep crater using Figure 4.3 for a range of explosive yields.

Table 2.1

Explosive Yield (kt)	Scaled Crater Depth	Scaled DOB	DOB (ft)	DOB (m)
5	87.8	81.9	131.5	40.2
10	71.6	40.5	79.7	24.3
15	63.6	31.1	69.0	21.0
20	58.4	25.0	60.3	18.4
100	36.4	8.3	32.2	9.8
765	20	0	0	0

The last row shows that for a true surface burst (albeit where the device is typically half-buried, NOT resting on the surface) with DOB = 0, it would take an explosive yield of 765 kt to blast a 43-meter crater!

Even 5 kt precludes any consideration this explosion could be of chemical origin. The largest planned conventional explosion on record—Minor Scale (June 1985)—with 4744 tons ANFO (4.2 kt TNT equivalent) required a hemisphere 44 feet in radius (see photo below). Now imagine that at DOB=131.5 ft!

 

Fledgling MSM Attempts to Downsize Crater Depth

Although my lead tweet in this thread ended up garnering overwhelmingly positive replies, nearly 2K retweets and 3K likes, all those so-called alternative media #SCAM who had been following me for months or even years completely ignored it. And so did the Ziotrolls. And so did the “fact checkers” and all their supposed “experts” who had done so much to refute the whole idea that Beirut was nuked. I was waiting to take on anyone who wanted to challenge my calculations but, to my surprise, no one did. I’d like to think that the “fact checkers” knew that a 43-meter crater depth was a “nuclear smoking gun” and had no way to refute my calculations.

There was, however, a fledgling effort to radically downsize the depth of the crater. On August 11, the Washington Post published an article by Middle East correspondent Sarah Dadouch titled “At Beirut’s shattered port, a crater nearly 50 yards deep and small signs of previous live.” ⁹

Dadouch does not report the source for her info on the crater’s depth but she did interview a Lt. Andrea (who “declined to give his last name in line with French security protocol”), the leader of the 63-member French search-and-rescue team, who must surely have been involved with the “French experts on the scene” that had told the anonymous “Lebanese security official” that the crater was 43 meters deep. One supposes Dadouch could have just been repeating the 43-meter crater depth reported by AFP—which would be equal to approximately 47 yards, close enough to report “nearly 50 yards deep”—but it is possible she heard it directly from Lt. Andrea.

 

However, a couple of days later, the Washington Post suddenly changed the headline, downsizing the crater from 50 yards to 15 yards deep although the URL remained the same, retaining the text “a-crater-nearly-50-yards-deep” of the old title. ¹⁰ 

As to why the Washington Post downsized the crater, all the paper had to say was “Correction: Earlier official estimates of the depth of the bomb crater have been revised downward and the story has been corrected to reflect that the blast left a crater of just over 15 yards in depth.“

I even tweeted a joke when I saw the article suggesting the Washington Post was on the run from my cratering analysis!

BTW @washingtonpost an actual 1.2-kT bomb resting on the surface (HOB=3.5 ft) still only blasted a crater 17 ft deep, nowhere near 15 yards.https://t.co/3ggfkyOVAN

— Bruce Baird (@drbairdonline) August 15, 2020

The only other #MSM source that I have found that reported this downsized 45-foot deep crater was Nabih Bulos at the Los Angeles Times who nonchalantly opened this August 18th article “To understand the catastrophic destruction wrought by the Aug. 4 detonation of 2,750 tons of ammonium nitrate in Beirut’s port, don’t look at the 45-foot-deep crater at the explosion’s epicenter. Instead, go a third of a mile to the east.” ¹¹

‘Everything has been pulverized,’ but cleanup and investigation of Beirut blast press on

For some reason, this effort to downsize to a 45-feet deep crater idea seemed to have died on the vine as not even Wikipedia, who might have otherwise been expected to following the lead of the Washington Post, did not pick up on this 45-foot deep crater, retaining their original report of a 43-meter deep crater.

There was one later equally fledgling effort to downsize the depth of the Beirut crater even more to 4-6 meters.

In an article published in the French daily L’Opinion, Jean-Dominique Merchet reported “This crater is not 43 meters deep, as we have read in various media, but ‘four to six meters’ in water, assures Lieutenant-Commander Aymeric Barazer, who commanded the detachment of French clearance divers dispatched to Beirut.” ¹²

The information was picked up the next day (September 7) by http://www.arretsurimages.net. ¹³

 

And the day after that (September 8) Wikipedia changed the section on crater dimensions to read “Within the port area, the explosion destroyed a section of shoreline and left a crater roughly 124 m (407 ft) in diameter and 4 m (13 ft) to 7 m (23 ft) in depth,” adding a citation to the www.arretsurimages.net article to the earlier citations. It is unclear why Wikipedia reported 4-7 meters rather than 4-6 meters. ¹⁴

Curiously a mere 5 days later (September 13), Wikipedia restored the original 43 meter depth without any explanation or discussion but kept the http://www.arretsurimages.net citation.

Even though the crater is filled with water, there is really no reason for this insane range of expressed opinions about the depth of the Beirut crater from #MSM sources. It sure appears that the Lebanese and French authorities went to great lengths to obscure the information about depth. A small survey boat equipped with an echosounder could have easily mapped the entire crater and made it available for all to see just like the satellite maps that were used to determine the crater diameter. Take a look at this short video titled “How to conduct a Bathymetric Survey”: ¹⁵

So why weren’t these boats on top of the water-filled crater at the port of Beirut in the days after the explosion equipped with an echosounder? Or were they and we just haven’t seen what they found yet? ¹⁶

 

Here's a fascinating video from Aug 9 of Beirut's Ground Zero showing how truly big the sea-filled crater was. You can also see divers & rafts that I think may be the "French experts" who actually measured the 43-m crater depth that was also reported Aug 9https://t.co/RsvKLz7R4P

— Bruce Baird (@drbairdonline) September 5, 2020

 

Working with Crater Diameter

Even if we had no exact measurement of apparent crater depth, we can make the case that only an underground nuclear explosion could have blasted such a large crater, based solely on the diameter of the crater as determined by the analysis of satellite images of the port of Beirut. We have already noted that Bellingcat on August 4th calculated a diameter of 150 meters. CNN reported 124 meters on August 5th, a measurement that was adopted as “official” by Wikipedia (along with the 43-meter depth reported by AFP). Also on August 5th the New York Times put the diameter at 140 meters (see image below).

Since #MSM sources do not precisely agree on the exact measurement for the crater diameter, we will work with a range of estimates from 120-150 meters.

To make the case that the measured crater diameter supports the hypothesis that the port of Beirut was nuked on 4 August 2020, we can show a range from 120-150 m for the crater diameter is actually consistent with a 43-m deep crater.

Crater depth and diameter are correlated. Cratering studies have shown the crater radius-to-depth ratio increases smoothly for the same size explosive yield as DOB increases from a surface burst down to “optimum DOB.”  It is easy enough to show that the largest empirical radius-to-crater ratio (and thus the shallowest crater depth) that could be expected is still deep enough that it would have been impossible with conventional (non-nuclear) explosives. 

We have already discussed re Jangle Sugar, Jangle Uncle, and Teapot ESS that as DOB increases, the crater depth also increases up to the point of the “optimum DOB.” Actually the entire crater profile changes from the largest radius-to-depth ratio for a surface blast to the smallest radius-to-depth ratio at the “optimum DOB.” Based on the data in Table 2,  Jangle Sugar (HOB=3.5 ft) had a crater radius/depth (R_a/D_a) ratio of 2.65, equal to a crater diameter/depth ratio of 5.3. Jangle Uncle (DOB=17 ft) had a diameter/depth ratio of 4.9 and Teapot Ess (DOB=67 ft) 3.2. Thus for the same 1.2 kt device, we have a range of 3.2-5.3 for crater diameter-to-depth ratio. Two other widely different nuclear tests for which we have specific information fall within this range of crater diameter-to-depth ratios: 4.1 for Johnnie Boy (.5 kt @ DOB=1.75 ft) and 3.7 for Storax Sedan (100 kt @ DOB=635 ft).

Based on a 3.2 to 5.3 range in the ratio of apparent crater diameter to depth and a range of estimates for the diameter of the 2020 Beirut crater of 120 to 150 meters, we can estimate the crater depth as being between 22.6 meters minimum to 46.9 meters maximum. Although a 43-meter crater depth certainly lies in this range, the point here is that to blast the shallowest crater (22.6 meters deep) with a surface blast (DOB=0) would require an 85.9 kiloton explosion which would necessarily be nuclear.

 

The Sedan Event

When I tweeted my original analysis of Beirut crater back in August 2020, I lamented that I did not have any figure comparable to Figure 4.3 for crater radius rather than crater depth. The booklet Operation Sun Beam, Shots Little Feller I and II: Crater Size and ShapeI (1965) in which I found Figure 4.3 did list in its Table of Contents Figure 4.4 “Scaled crater radius versus HOB (DOB) for large explosions in desert alluvium and similar media” as well as Table 4.1 “Cratering Data for NE and Comparable HE Explosions in Desert Alluvium and Similar Media” from which we could plotted Figure 4.4. But both are still for some reason classified and thus deleted from the booklet. Indeed, seeing the kind of information that was deleted from this booklet and other government documents on cratering when they were declassified, it is rather amazing that Figure 4.3 was NOT deleted!

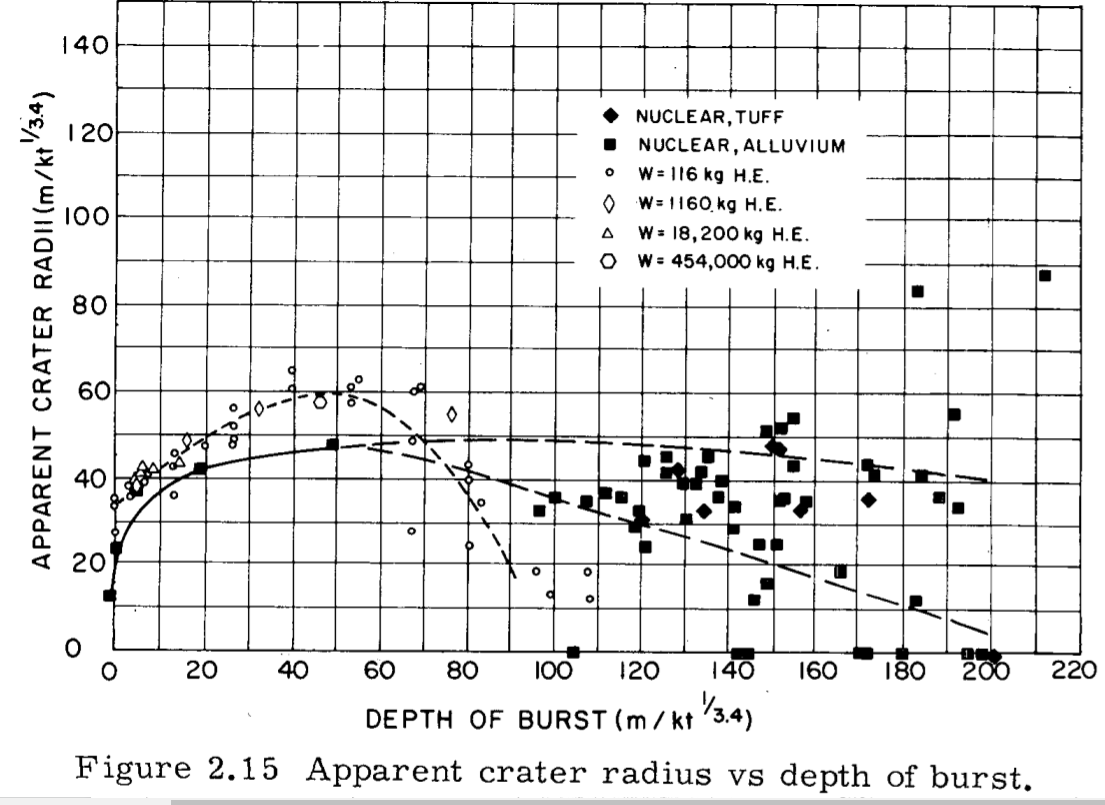
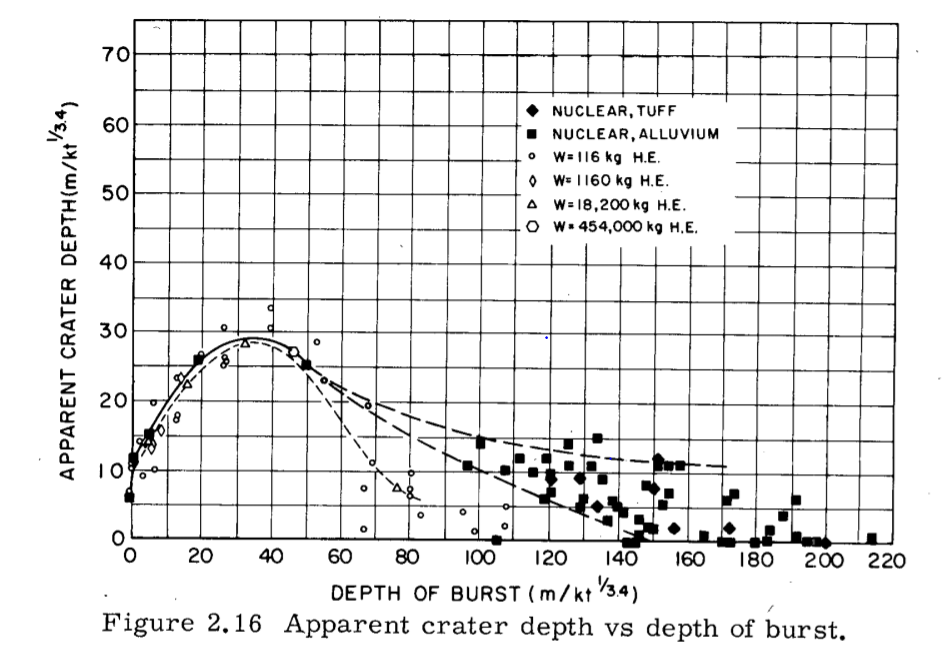
Thus I was pleasantly surprised to learn (from the work of Peter Goldstein discussed below) of another government document that came out in the very same year as Operation Sun Beam, Nordyke & Williamson’s The Sedan Event (1965) that contained figures for both scaled apparent crater depth (Figure 2.16) and scaled apparent crater radius (Figure 2.15), for both chemical explosives (HE) and nuclear explosives (NE). ¹⁷

Working with Figure 2.15 “Apparent crater radius vs depth of burst” is a lot like working with Figure 4.3 except Figure 2.15 is for scaled apparent crater radius while Figure 4.3 was for scale apparent crater depth.

The short-dash curve on the left is “a curve fit to the 116-kg (256-lb) data by an inverted data regression analysis” while the solid curve on the left is a curve fit to the sparse nuclear data.

Figure 2.16 is a similar plot to Figure 2.15 except for scaled apparent crater depth, like Figure 4.3.

Nordyke & Williamson noted the lower scaled apparent crater radii for nuclear compared to HE explosives and concluded the difference was due, not to different scaling, but to “the reduced efficiency of nuclear explosives for apparent crater radii”. However, the close similarity of chemical & nuclear explosive for scaled apparent crater depth suggests if anything an increased efficiency of nuclear explosives for apparent crater depth.

As might be expected, my Table 2.2 DOB calculations for an apparent crater depth of 43 meters using both dashed HE line for 116-kt (256-lb) and the solid NE line from Figure 2.16 matches fairly closely the results (we have already seen in my Table 2.1) based on Figure 4.3.

Table 2.2

Explosive Yield (kt)	43-m deep crater DOB (Fig. 4.3) (m)	43-m deep crater DOB (Fig. 2.16 HE) (m)	43-m deep crater DOB (Fig. 2.16 NE) (m)
5	40.2	37.6	33.9
10	24.3	28.5	27.0
15	21.0	26.6	22.8
20	18.4	21.7	20.0
100	9.8	8.1	4.3

 

The one major difference between Figure 4.3 and Figure 2.16 HE curves comes as you approach HOB = 0. Using Figure 4.3, I calculated that at DOB = 0, a 43-meter deep crater would have required an explosive yield of 765 kilotons TNT equivalent. The figure 2.16 NE curve gives a similar result. However, based on Figure 2.16 HE curve, the yield @ dOB = 0 is only 142.5 kilotons.

Both Figure 4.3 and Figure 2.16 NE curves show the scaled crater depth dropping dramatically as  the scaled DOB moves closer to HOB = 0, whereas N&W’s linear regression line for Figure 2.16 HE shows a much more gradual decline. N&W’s Figure 2.2 shows even more clearly that the HE line intersects the y-axis at 10 m/kt^1/3.4 whereas the Figure 2.16 NE line intersects at about 6 meters, quite close to the 20 feet observed in Figure 4.3.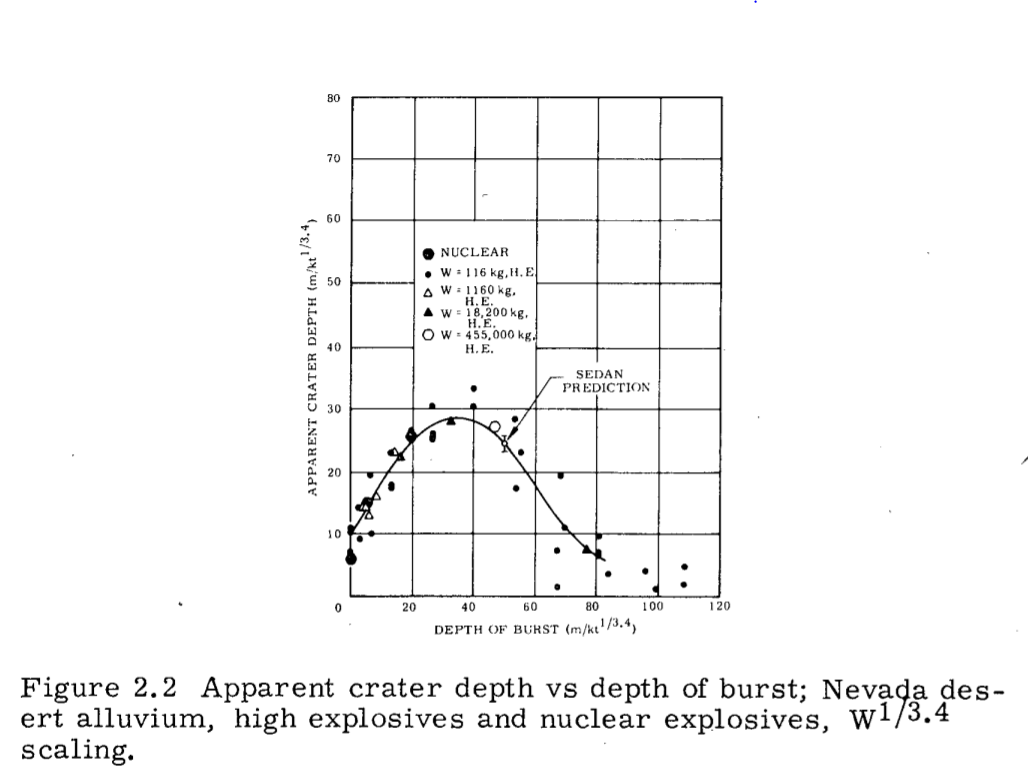

Since Figure 2.15 for scaled crater radius shows the HE line dropping only gradually and NE line dropping dramatically as scaled DOB moves closer to HOB = 0, we might expect DOB calculations based on scaled crater radii to also show a sharp divergence between HE and NE @ DOB = 0.

Interestingly, we find using the Figure 2.15 HE curve that any device greater than 10 kilotons detonated just below the surface would have blasted a crater wider than 150 meters diameter. In other words, any device greater than 10 kilotons would have to have been detonated aboveground in order to blast a crater less than 150 meters wide.  However, a 5-kt HE @ DOB = 24.1 meters and a 10-kt HE @ DOB = 8.5 meters would have produced a 150-meter diameter crater. As for a 120-meter diameter crater, even a 10-kt HE device would have to be aboveground but a 5-kt HE device @ DOB = 7.5 meters would have done the job.

Using the Figure 2.15 HE curve to calculate the apparent crater diameter, if a 5-kt HE device had been detonated @ DOB = 37 m (the DOB that would have been sufficient to blast a 43-m deep crater according to Figure 2.16 HE curve), we find the 5-kt HE device would have created a 165-m wide crater, 15 meters wider than the largest published measurement (Wired’s 150-m diameter).

Based on Figures 2.15 HE and 2.16 HE curves, the limiting factor in terms of choosing a combination of explosive yield and DOB is that placing any device deep enough to blast a 43-meter deep crater produces a crater much wider than 150 meters while placing the device shallow enough to limit the crater diameter to 150 meters or less produced a crater significantly less than 43 meters. A 5-kiloton device with a DOB between 20 meters and 40 meters comes closest to meeting both parameters. At DOB = 40 meters, a 5-kiloton device would blast a sufficiently deep (44.5 m) but excessively wide (167 m) crater. At DOB = 20 meters, the same 5-kiloton device would create a sufficiently wide (145 m) but too shallow (32.1 m) crater. Thus, if we have greater confidence that the width of the crater was no more than 150 meters, the Figures 2.15 HE and 2.16 HE curves suggest the crater depth was somewhat less than the reported 43 meters.

However, using these HE curves raises the problem we have noted already, thinking that this explosion was caused by the detonation of chemical explosives equivalent to 5-kt TNT 20-40 meters below the surface is totally unrealistic. Repeating once again, the largest planned conventional explosion on record—Minor Scale (June 1985)—with 4744 tons ANFO (4.2 kt TNT equivalent) required a hemisphere 44 feet in radius (see photo below). Now imagine that at 20-40 meters below the surface!

Furthermore, if anything, the difficulty in trying to find a solution to the explosive yield of the 2020 Beirut explosion using Figure 2.15 HE and Figure 2.16 HE based on 116-kg (256-lb) data should lead us to suspect that these curves are likely inappropriate for analyzing the Beirut explosion. However, using the Figure 2.15 NE and 2.16 NE curves, we have no such problems as faced with the HE curves. 

Using the Figure 2.15 NE curve, Table 2.3 shows the required DOB for various explosive yields to blast a range of apparent crater diameters: 120, 130, 140 and 150 meters.

Table 2.3

Depth of Burst (DOB) for Various Crater Widths & Various Yields

Explosive Yield (kt TNT equiv)	150-m wide crater DOB (Fig. 2.15 NE) (m)	140-m wide crater DOB (Fig. 2.15 NE) (m)	130-m wide crater DOB (Fig. 2.15 NE) (m)	120-m wide crater DOB (Fig. 2.15 NE) (m)
5	59	37	25	17
10	32	15	12	7.3
15	30	10	7.1	5.8
20	26	7.7	6.3	2.7
100	0.0	-0.3	-0.6	-0.9

The negative numbers represent DOB above the surface, e.g., DOB = -0.3 is the same as HOB = +0.3.

Comparing the data from Table 2.2 (based on Fig. 2.16 NE curve) with the data from Table 2.3 (based on Fig. 2.15 NE curve), the calculations show that for a 5-kt device @ DOB = 33.9 m (i.e., deep enough to blast a 43-m deep crater), the apparent crater diameter would be in the range 130-140 meters, while for 10-kt @ DOB = 27.0 m, 15-kt @ DOB = 22.8 m and 20-kt @ DOB = 20.0 m the crater diameter would be in the range 140-150 m meters. A 100-kt @ DOB = 4.3 m would blast a crater over 150 meters in diameter.

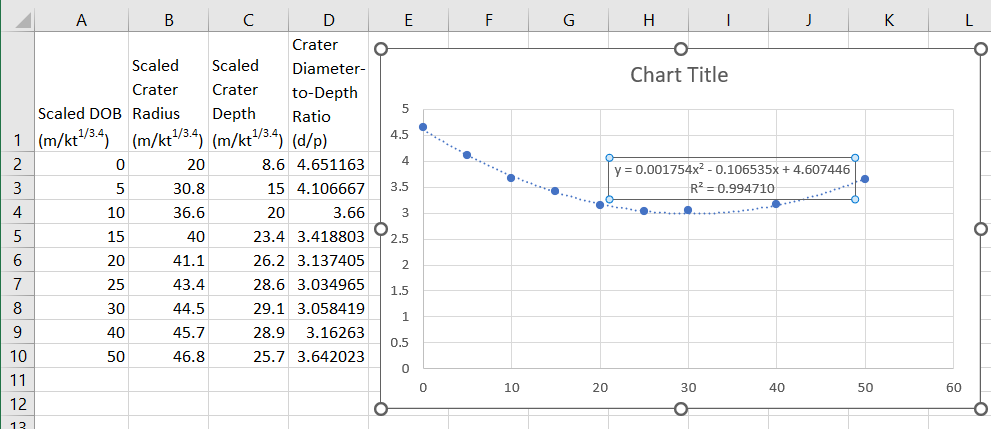
An alternative way to use the data from Figs. 2.15 NE and 2.16 NE curves that can yield more precise estimates of both charge and DOB is to derive a third curve for that ratio of scaled apparent crater diameter to depth as a function of scaled depth of burst. The best-fit equation is

Diameter-to-Depth Ratio =  0.001754 (Scaled DOB)^2 – .1065 (Scaled DOB) + 4.6074 (1)

 

 

We can see from the graph of this best-fit equation, the apparent crater diameter-to-depth ratio varies from a maximum of 4.61 @ DOB = 0 to a minimum of 2.99 @ scaled DOB = 30, somewhat smaller ratios than we observed from the measured ratios for Jangle Sugar, Jangle Uncle, and Teapot ESS.

Since the ratio of “scaled” apparent crater diameter to depth is identical to the ratio of “unscaled” apparent crater diameter to depth, if we can solve this equation using the measured apparent crater diameter to depth ratio to calculate the scaled DOB, and thence the actual charge and DOB.

Since there is still some uncertainty in the measurement of diameter and depth, as an example, we will assume an apparent crater depth of 43 m and make calculations for a range of apparent crater diameters (120, 130, 140 and 150 m). Since the equation is quadratic we will use the quadratic formula which will sometimes give us two possible answers that satisfy the equation, (1) a shallower, more powerful explosion and (2) a deeper, less powerful explosion.

Table 2.4

Calculated Charge and DOB for Apparent Crater Depth = 43 m

Apparent Crater Diameter (m)	Apparent Crater Radius (m)	Apparent Crater Diameter-to-Depth Ratio	Scaled DOB (calc. using Eq. 1)  (m/kt1/3.4)	Scaled Apparent Crater Radius (m/kt1/3.4)	Charge (kt TNT equivalent)	DOB (m)
120	60	2.79	N/A	N/A	N/A	N/A
130	65	3.02	26.0 34.7	43.5 45.3	3.92 3.41	39.2 49.5
140	70	3.26	18.05 42.7	40.9 45.8	6.22 4.23	30.8 65.4
150	75	3.49	13.5 47.2	38.9 46.1	9.32 5.23	26.0 76.8

 

The results from Table 2.4 show that for an apparent crater depth of 43 m, an apparent crater diameter of 120 m is impossible using Equation 1 because the apparent crater diameter-to-depth ratio (2.79) lies below the curved line. 

Using this apparent crater diameter-to-depth ratio approach also suggests that an underground nuclear explosion as low as 3.5 kiloton TNT equivalent (lower than the minimum 5 kt suggested in the August 2020 thread) could have blasted the 2020 Beirut crater and the maximum explosive yield was less than 10 kt. 

However, once again we note that there is ABSOLUTELY NO WAY that the explosion of 2,750 tonnes of ammonium nitrate sitting on the floor of a warehouse in the port of Beirut could possibly have blasted such a huge crater. Only a nuclear device could have done so.

The uncertainty in measures of both crater depth and diameter as discussed in this chapter, as well as uncertainty about the exact nature of the nuclear device employed in Beirut and the applicability of correlations derived empirically from tests carried out in the Nevada Testing Site in the 1950s & 1960s to this particular device employed in the particular conditions of the Port of Beirut in August 2020, preclude making any more precise statement of the specific yield and depth of burst (DOB) of the explosion beyond saying it was an underground multi-kiloton nuclear device. However, I did end my twitter thread with the thought of how dastardly it would have been if the perpetrators of the 2020 Beirut explosion had used a 15-kiloton nuke, the same yield that exploded over Hiroshima in “honor” of the 75th anniversary.

How dastardly if the perps used a 15 kT MRR in Beirut in “honor” of 75th Hiroshima anniv! Buried 69 ft below surface it could have blasted the 43-m deep crater. And if the perps used an MRR in Beirut, what are the odds they have used them before – and will use them again?
45/

— Bruce Baird (@drbairdonline) August 14, 2020

 

The So-Called “Experts” & Cratering Analysis

So far #MSM, #SCAM, “fact checkers,” and so-called “experts” have all pretty much ignored any kind of cratering analysis, perhaps because the #Gatekeepers know that any rigorous cratering analysis would destroy the “official” story and raise again the whole “nuclear” question that they tried to bury on August 4th.

As of February 2021, I know of only two “experts” who have even attempted to address whether the crater is consistent with the official” story and, as we will see, a critique of their work will show the flaws in their assumptions, calculations and conclusions. 

As far as I know, the only #MSM attempt to address the issue of whether the “official” story could explain such a large crater was a Wired article “The Tragic Physics of the Deadly Explosion in Beirut” that was published online on August 6th. ¹⁸

 

I was informed of the Wired article on the same day I started my twitter thread by some troll @LooseSid who thought this article completely refuted my arguments (that I am certain he or she hadn’t read because I had only started posting them) and wanted me to retract, as they called it, my so-called “conspiracy BS.”

I suggest you read this, then retract your conspiracy BS:https://t.co/ys9MPSQoHm

— LooseSid🇷🇺🇵🇸🇿🇦 (@LooseSid) August 9, 2020

The Wired article was written by Rachel Lance who describes herself as “a biomedical engineer with a doctorate in the patterns of injury and trauma that follow an explosion” that I guess would qualify her as an “expert” in #MSM circles as long as she is there to defend the “official” story.

The cratering analysis in the Wired article is restricted to one paragraph:

I could not agree more with Lance’s line opening line in this paragraph: “Thanks to modern technology that charge size can be calculated scientifically too, even while waiting for more complete information to trickle out, using the size of the telltale crater”. Unfortunately for Lance “the size of the telltale crater,” far from supporting the “official” story as she thought, is actually “the nuclear smoking gun”!

Lance links “charge size can be calculated” to a Google Books preview of Gilbert F. Kinney and Kenneth J. Graham’s Explosive Shocks in Air, 2nd ed.(1985).

 

Uncontroversially, Lance then claims that “Analysis of the aerial photographs of the pier shows a crater in the range of 120 to 140 meters in diameter.”

Then, Lance turns to Kinney & Graham in order to assert

blast physics mixed with history tell us that to carve a chunk that size from the side of the planet requires a charge equivalent to 1.7 to 5.4 million kilograms of TNT (that’s 3.8 to 11.8 million pounds for any Americans dragging their feet on converting to metric).

Actually, Graham & Kinney does not have much at all on cratering, just a brief section found on pages 9-10: ¹⁹

 

For her calculation, Lance employs equation (1-4) which Kinney & Graham explain thus: 

“A statistical study of perhaps two hundred accidental large explosions provides an equation for crater diameter d in metres in terms of explosion yield W in kilograms TNT, and for explosions on the surface, as

d = 0.8W^1/3               (1-4)

For the 2020 Beirut crater diameter in the range 120 to 140 meters, Lance calculates for d = 120 meters, W = 1.7 million kilograms of TNT (3.8 million pounds and for d = 140 meters, W = 5.4 million kilograms of TNT (11.8 million pounds).

There are a couple of problems with Lance’s presentation.

First, she miscalculates d = 120 meters, which is actually 3.4 million kg TNT, NOT 1.7 million kg TNT.

Second, she does NOT report the results in equivalent tons of TNT which is generally the way the explosive yield is reported for large explosions. For d = 120 meters, W is 3.7 kilotons of TNT and for d = 140 meters, W is 5.9 kilotons of TNT!

It is no wonder this first cratering analysis of the 2020 Beirut explosion was completely ignored by the “fact checkers” and other so-called “experts”! Since the “official” story reported the explosion was caused by 2.75 kilotons of ammonium nitrate that—even if it could dubiously be made to explode like a bomb lying on the floor of an open warehouse—was not nearly as explosive as TNT. Thus Lance’s number of 3.7 kt for 120-m wide crater or 5.9 kt for 140-m crater would simply be too great to be explained by the “official” story.

Indeed, as already noted, the New York Times reported the explosive yield of the ammonium nitrate was only 1.155 US kilotons TNT equivalent. So WHERE is all that extra explosive power to blast that wide of a crater coming from if not ammonium nitrate? Lance didn’t address that question and the “fact checkers” and “experts” simply ignored her cratering analysis.

If we stepped up the rigor of Lance’s cratering analysis, the discrepancy in explosive force between the “official” story and “the telltale crater” becomes even worse for the “fact checkers” and “experts.”

However standard a textbook Kinney & Graham might be, Equation 1-4 is rather suspect. They say it is based on “a statistical study of perhaps two hundred accidental large explosions” but they never cite this statistical study anywhere. Don’t we need a chance to see the evidence upon which Equation 1-4 is based?

Furthermore, Kinney & Graham claim Equation 1-4  is valid for “explosions on the surface.” But what exactly do they mean by “on the surface”? Since they reference “accidental large explosions” one might imagine they mean cased where explosives are actually stacked up on the surface of the earth. But that is NOT what cratering analysts mean when they refer to a “surface explosion.” When cratering analysts refer to a “surface explosion” they mean very specifically one where the CENTER of the explosion is at the surface, i.e., HOB = 0. This typically involves half-burying the explosive device in the ground so only the top half is exposed, I doubt seriously whether ANY of the explosives in the 200 accidental explosions were half-buried!

If the explosives are resting on top of the ground, then HOB is absolutely NOT zero. Rather HOB is determined by the height of the center of the explosion above the surface regardless of whether the explosives themselves are in contact with the surface or not.

A classic example is Jangle Sugar. As we have already noted, Jangle Sugar was designed to be a test of a surface bomb (“Sugar” was mnemonic for “surface). However, when the analysts studied the test, they realized that the 7-foot tall nuclear device was not half-buried as it should have been but actually resting on the ground so the HOB was actually half of the height (HOB = +3.5 feet) of the device. Thus the first supposed surface nuke was actually an aboveground nuke!

All the images of stacks of bags of ammonium nitrate sitting on a floor in a warehouse in the port of Beirut show clearly that, if all these bags could seriously be considered an “explosive”, HOB would hardly be zero. Rather, assuming the man standing next to the bags is about 6 feet tall, we would have to take half of the height of the stack to be approximately 3 feet.

 

The New York Times depicted what the inside of the warehouse looked like with all the bags of ammonium nitrate stacked up. ²⁰

Although 3 feet may seem insignificant when we are talking about the explosion of a 1.155 kt “bomb,” cratering analysts recognize that there is world of difference between a surface explosion (HOB = 0) and an aboveground explosion (HOB = 3 feet) in terms of cratering. Indeed, Kinney & Graham recognize that “Diameter of the crater from an explosion also depends on the location of the explosion relative to surface level. Thus explosions above a surface may not create any crater at all.“

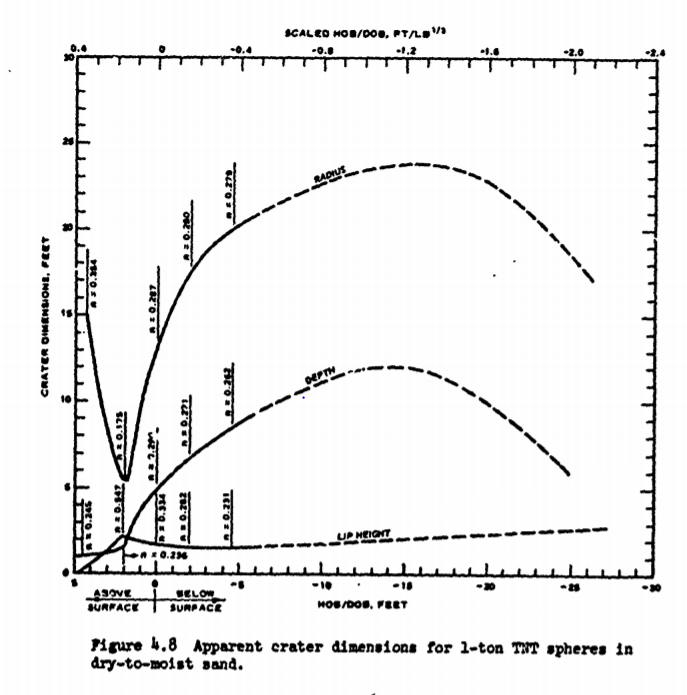
Figure 4.8 “Apparent crater dimensions for 1-ton TNT spheres in dry-to-moist sand” taken from Cratering by Explosions: A Compendium and an Analysis (1974) produced by the U. S. Army Engineer Waterways Experiment Station tells a more precise story than Kinney & Graham. ²¹

Figure 4.8 shows that as you move left from HOB = 0 increasing to HOB = 2 feet, the apparent crater radius drops dramatically from 13 feet to near 5 feet while the crater depth drops from 5 feet to near 1 feet. At HOB > 2 feet, the 1-ton TNT spheres do not even blast a conventional crater; rather they produce a “compression crater” where the volume of the apparent crater is “largely attributable to the permanent compression of the underlying medium” Furthermore, as can be seen in Figure 4.8,  with a compression crater, as the HOB increases, the radius increases dramatically but the depth hardly changes.

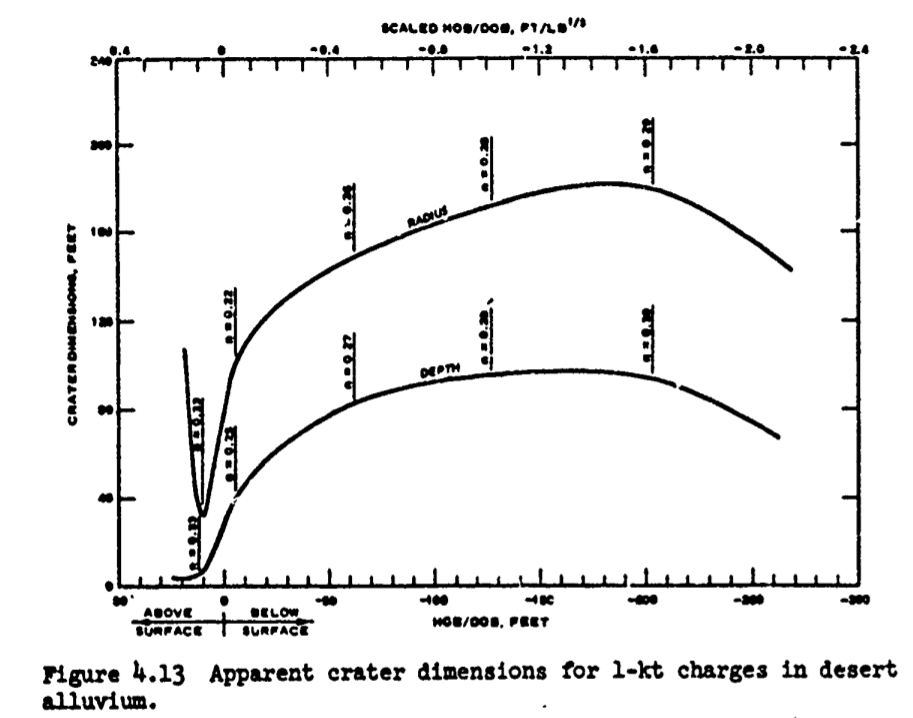
The same pattern of the impact of HOB > 0 can be found for 1-kt charges in desert alluvium if Figure 4.13 where the apparent crater radius drops rapidly from 73 feet @ HOB = 0 down to 33 feet @ HOB = 10 feet and the apparent crater depth from 31 feet down to 7.8 feet.

If Kinney & Graham are not correcting for the height of the explosives above the ground, this could explain why Equation 4.1 gives results for apparent crater radius that are far lower than other studies like that captured by Figure 2.15. Using the Figure 2.15 He curve, we can determine that for HOB = 0, a 120-meter diameter crater in desert alluvium would require 6.25 kt TNT equivalent and 140-meter diameter crater 10.6 kt, both almost double the amount calculated by Lance from Equation 1-4.

But the main point here is that, whether one accepts Kinney & Graham’s Equation 4.1 or Figure 2.1, the resultant explosive yield for the 2020 Beirut explosion is far greater than can be accounted for by 2,750 tonnes of ammonium nitrate.

As an aside, Lance dismisses the possibility that “nuclear weaponry” could have been used to blast the 2020 Beirut crater on the amazingly ignorant grounds that “nuclear weaponry is set to detonate several hundred feet above ground level, and therefore doesn’t exert enough force directly on the soil to create a crater,” as if “nuclear weaponry” cannot detonate at or below the ground!

As of February 2020, the only other effort by a so-called “expert” to perform cratering analysis on the 2020 Beirut explosion were two papers by Peter Goldstein (PhD Physics, UC Santa Barbara), a physicist at Lawrence Livermore National Laboratory (LLNL) since 1989.

Goldstein’s first attempt to use cratering analysis was an LLNL paper  “Beirut Explosion Yield and Debris Cloud Height – The Effect of Media Saturation and Harbor Proximity,” published online on 17 August 2020. ²²

 

In his August 2020 paper, Goldstein used cratering analysis to estimate “the yield of the August 4th, 2002 Beirut explosion to be approximately 2.3 kilotons, with a range between 1.7 and 3.4 kilotons.”

However, what he seemed most interested in was not so much determining the actual explosive yield as figuring out what adjustments he had to make to the cratering analysis in order to come up with a number that could plausibly be explained by the “official” story of 2.75 kt of ammonium nitrate.

One would have hoped that a physicist from LLNL would have addressed the implausibility of 2.75 kt of ammonium nitrate lying on the floor of warehouse could have even exploded or ever have blasted a crater. But instead he puts on the mantle of “expert” whose job is always to shore up the “official” story, not question it.

Unfortunately for Goldstein’s dreams of offering his “expertise” on behalf of the “official” story, @ain92ru quickly observed that the author had amazingly used crater diameter rather than radius in his calculations.

Am I the first one to notice that the author confused crater radius with diameter?
This mistake is very easy to make, and everyone has lost 1/2s in physic formulae many times, but seriously, when he got cloud height two times higher than in reality, it should have rang a bell pic.twitter.com/iu8qhQfCsZ

— Ilya A. (@ain92ru) August 22, 2020

Although this obvious radius error would likely have sealed the fate of Goldstein’s paper, it is interesting that it was not the chief concern of the other “experts” who were on Twitter discussing the article. No, their biggest concern was that Goldstein’s estimate of 2.3 kt for the explosive yield of the 2020 Beirut blast was TOO HIGH.

“Expert” Vipin Narang, Associate Professor of Political Science at MIT, former chemical engineer, and author of Nuclear Strategy in the Modern Era (2014), in response to Goldstein’s “preferred” 2.3 kt, tweeted “That yield estimate seems wildly high, like theoretically improbable for 2750 tons of NH4NO3, and an order of magnitude higher than the likely observed yield. Not sure I buy this.” ²³

Similarly “expert” Jeffrey Lewis—who we have already met in Chapter 1 as denying that Beirut was nuked because “There are literally none of the phenomena one sees with a nuclear explosion”—replied re Goldstein’s 2.3 kt that “I don’t think any of us buy it!”

I don’t think any of us buy it!

— Dr. Jeffrey Lewis (@ArmsControlWonk) August 22, 2020

You see “expert” Jeffrey Lewis way back on the day of the Beirut explosion on August 4th had already amazingly concluded that the explosion was only 275 tons TNT equivalent so no wonder he was so dismissive of Goldstein’s 2.3 kt. 

Fun fact! Ammonium nitrate varies greatly in TNT equivalency, from 3% to 56%, depending on the details. If it was TGAN, 275 tons would be about right. pic.twitter.com/BRZkW1pFXr

— Dr. Jeffrey Lewis (@ArmsControlWonk) August 4, 2020

 

“Expert” Martin Pfeiffer—who we have also already met in Chapter 1—said Goldstein’s 2.3 kt was “patently wrong and I bet there is an early math error.” 

this is patently wrong and I bet there is an early math error.

— Martin “Doomsday” Pfeiffer 🏳️‍🌈 (@NuclearAnthro) August 22, 2020

If Pfeiffer would have looked through the other replies, he would have seen that @ain92ru had two hours earlier pointed out that Goldstein had indeed made “an early math error” mistaking crater diameter for radius.

I’m sure all the “experts” thought, ahh, rookie mistake! How foolish of Goldstein! The explosive yield must have been half of what Goldstein was reporting, something like 1.15 kt. Cased closed.

Nobody bothered to look for any other “mistakes” in the paper. I am confident that if it hadn’t been for the rather obvious radius faux-pas that Goldstein’s paper would have been totally accepted and gotten a mention in the “official” Wikipedia article on “2020 Beirut explosion”! ²⁴

But, whether it was the radius fiasco and/or something else, by August 25, the day after I had printed Goldstein’s August report, it was scrubbed from the internet except for a bibliographic entry maintained on the Wayback Machine and a few tweets. ²⁵

You might think that Goldstein might have wanted to just go ahead and hide his head in shame and never go anywhere near crater analysis ever again.  No fear! Two months later (25 October 2020), he published a new & improved paper with a quite similar sounding title “Beirut Explosion Yield and Mushroom Cloud Height – Effects of the Near Source Environment.” ²⁶

In the October 2020 paper, Goldstein downsized the preferred explosive yield to 1.4 kt TNT equivalent, with a lower bound of 0.7 kt and upper bound of 2.75 kt.

As far as I can tell, this second paper from Goldstein has been almost totally ignored by the “fact checkers” and “experts” who apparently don’t think the “official” story needs shoring up anymore, at least not from cratering analysis.

Although it may seem like beating a dead horse to devote much attention to an author whose first paper was completely panned and withdrawn within a few days and whose second paper was completely ignored, papers that contain numerous other “freshman mistakes” (e.g., using the wrong exponent) along with making some highly questionable or erroneous assumptions, a closer look at Goldstein’s cratering analysis in his two papers is well worth a critique seeing as it is the only “serious” attempt by an “expert” to employ cratering analysis for the 2020 Beirut explosion.

A thorough analysis of Goldstein’s paper. at one level, shows how he biased his calculations in favor of coming up with acceptable numbers for explosive yield to support the “official” story. But, at another level, such an analysis provides insights not just into the approach of this particular “expert” but all “experts” when it comes to dealing with craters and large explosions and serves as a warning and template for understanding how “experts” will continue to distort “science” to obfuscate our understanding of such explosive events in the future, biasing “science” in favor of coming up with explanations that make no objective sense simply because they conveniently throw some support to the “official” story. When it comes to the 2020 Beirut explosion (and other events discussed later in this book), we should not trust the objectivity of any so-called “experts,” whether those who ignore or those who distort the evidence that runs contrary to the “official” lies they are determined to defend.

nHowever, because my thorough critique of Goldstein’s cratering analysis gets even more technical than the analysis already presented in this chapter, I will reserve the details of the critique for the Appendix to this chapter.